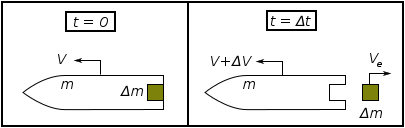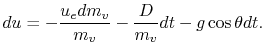+919674493673 
mailus@wbcsmadeeasy.in
Physics Notes For – W.B.C.S. Examination – The Rocket Equation.
পদার্থবিজ্ঞানের নোট – WBCS পরীক্ষা – রকেট সমীকরণ।
The Rocket Equation
We can now look at the role of specific impulse in setting the performance of a rocket. A large fraction (typically 90%) of the mass of a rocket is propellant, thus it is important to consider the change in mass of the vehicle as it accelerates.Continue Reading Physics Notes For – W.B.C.S. Examination – The Rocket Equation.
There are several ways to do this through applying conservation of momentum. Here we will apply the momentum theorem differentially by considering a small mass, 
, expelled from the rocket during time 
,
The initial momentum of the mass in the control volume (the vehicle) is 
. The final momentum of mass in the control volume (the vehicle and the mass expelled, ) is
 |
The change in momentum during the interval is
 |
 |
|
 |
since 
is a higher order term. Now consider the forces acting on the system which is composed of the masses 
(the rocket), and (the small amount of propellant expelled from the rocket during time ):
 |
Applying conservation of momentum, the resulting impulse, 
, must balance the change in momentum of the system:
![$\displaystyle m_v du - dm u_e = [(p_e - p_0) A_e - D - m_v g \cos\theta]dt.$](http://web.mit.edu/16.unified/www/SPRING/propulsion/notes/img1678.png){kind=small} |
Then since
 |
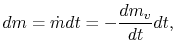where 
is the propellant mass flow rate, we have
![$\displaystyle m_v du = [(p_e - p_0)A_e + \dot{m}u_e - D - m_v g \cos\theta]dt,$](http://web.mit.edu/16.unified/www/SPRING/propulsion/notes/img1680.png){kind=small} |
or, for 
,
This is known as The Rocket Equation. It can be integrated as a function of time to determine the velocity of the rocket.
If we set 
, assume that at 
, 
, neglect drag, and set 
, then we can simplify the rocket equation to
 |
which can be integrated to give
 |
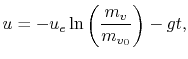where 
is the initial mass of the rocket. We can also write this result as
![$\displaystyle u = g \left[\textrm{Isp} \ln\left(\frac{m_{v_0}}{m_v}\right)-t\right].$](http://web.mit.edu/16.unified/www/SPRING/propulsion/notes/img1690.png) |
We can view this equation as being similar to the Breguet Range Equation for aircraft. It presents the overall dependence of the principal performance parameter for a rocket (velocity, 
), on the efficiency of the propulsion system (Isp), and the structural design (ratio of total mass to structural mass, since the initial mass is the fuel mass plus the structural mass and the final mass is only the structural mass).
Assuming the rate of fuel consumption is constant, the mass of the rocket varies over time as
 |
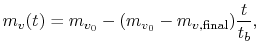where 
is the time at which all of the propellant is used. This expression can be substituted into the equation for velocity and then integrated to find the height at the end of burnout:
 |
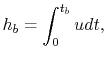which for a single stage sounding rocket with no drag and constant gravity yields
![$\displaystyle h_b = g \left[-t_b \textrm{Isp}\cfrac{\ln\left(\cfrac{m_{v_0}}{m_... ...}{m_{v,\textrm{final}}}-1\right)} + t_b \textrm{Isp} - \frac{1}{2}t_b^2\right].$](http://web.mit.edu/16.unified/www/SPRING/propulsion/notes/img1694.png) |
The final height of the rocket can then be determined by equating the kinetic energy of the vehicle at burnout with its change in potential energy between that point and the maximum height. This is left as an exercise for the reader.
Our own publications are available at our webstore (click here).
For Guidance of WBCS (Exe.) Etc. Preliminary , Main Exam and Interview, Study Mat, Mock Test, Guided by WBCS Gr A Officers , Online and Classroom, Call 9674493673, or mail us at – mailus@wbcsmadeeasy.in
Visit our you tube channel WBCSMadeEasy™ You tube Channel
Please subscribe here to get all future updates on this post/page/category/website
